Standard Deviation

Range and Normal Distribution

Variability

Variability reflects how scores differ from one another.  Variability can also be thought of as a measure of how much each score in a group of scores differs from the mean (average).  Together, variability and average, are used to describe the characteristics of a distribution.

​

​

​

​

​

 

Range

​

The Range  is the difference between the maximum value and the minimum value.  It tells how far apart scores are from one another in a distribution.

It is calculated by simply subtracting the lowest score in a distribution from the highest score.      r = h - l

• r is the range,

• h is the highest score,

• l is the lowest score

 

Example: 3, 3, 4, 5, 5        

r = 5 - 3

  r = 2

​

​

Standard Deviation

The Standard Deviation represents the average amount of variability in a score.  It is the average distance from the mean.

The larger the Standard Deviation, the larger the average distance from each data point is from the mean of distribution.

 

• It is calculated using the following formula:

​

​

​

​

​

http://mathematicalmusings.pressible.org/nhw2108/understanding-standard-deviation

 

​

Normal Distribution

Data can be "distributed" (spread out) in different ways.

​

​

​

​

​

        Spread more to left                     more to right    

 

 

 

 

 

or all jumbled

​

We say the data is "normally distributed":

​

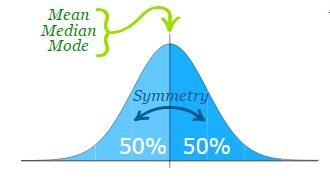
The Normal Distribution has:

• mean = median = mode

• symmetry about the center

• 50% of values less than the mean

• and 50% greater than the mean

​

​

​